There are 92 ways to place eight queens on a chessboard so that no queen is attacking any other. These fall into 12 equivalence classes. The 92 solutions are all rotations and reflections of these 12 basic solutions.
If you think about the previous numbers a minute, you might wonder why the total number of solutions is not a multiple of 12. There is one particular solution that is more symmetric than the others. So the total number of solutions 92 breaks down into
8 × 11 + 4 × 1.
To illustrate this, let’s look at two fundamental solutions: one that is particularly ordered and one that is particularly disordered in a sense that we’ll get to later on.
Most basic solutions, like the one below, are part of an equivalence class of eight solutions.
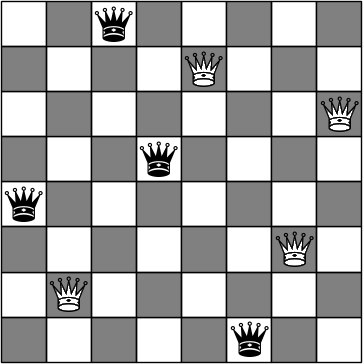
You can rotate the board 90° or reflect it about the middle[1], or some combination of both [2]. This amounts to eight possibilities.
This solution, however, is more symmetric.
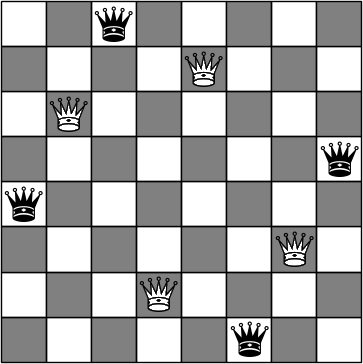
You can rotate the basic solution, but flipping it over does not create a new solution: a flip produces the same result as two 90° rotations.
It’s curious that there is only one highly symmetric solution to the eight queens problem. When I first saw the problem as a child I expected all the solutions to be highly symmetric. That may be why I wasn’t able to find a solution.
Among the 11 basic solutions that are less ordered, the one shown above is uniquely disordered in the following sense: no three queens lie on a straight line.
The eight queens problem is a problem about restricted straight lines. It says no two queens lie on the same rank, file, or diagonal. But if we look at all straight lines, then of course there is a line through any two queens. In the most orderly solution, every queen is on a straight line with two others. In the least orderly solution, no queen is on a straight line with two others.
In 1900 Henry Dudenay introduced the no-three-in-line problem, looking at ways to place points on a lattice such that no line goes through three points, with no restriction on the slope of the lines. So one family of solutions to the eight queens problem is also a solution to the no-three-in-line problem.
Related posts
[1] It doesn’t matter whether you flip about the horizontal or vertical axis.
[2] In fancy terminology, the action of the dihedral group D8 applied to a solution yields another solution.
The post Special solutions to the eight queens problem first appeared on John D. Cook.