I recently came across an upper bound I hadn’t seen before [1]. Given a binomial coefficient C(r, k), let
n = min(k, r − k)
and
m = r − n.
Then for any ε > 0,
C(n + m, n) ≤ (1 + ε)n + m / εn.
The proof follows quickly from applying the binomial theorem to (1 + ε)n + m.
I could imagine how non-optimal choice of ε could be convenient in some context, it’s natural to want to see how good the upper bound is for the best ε, which works out to be ε = n/m.
A little algebra shows this value of ε leads to
C(n + m, n) ≤ (n + m)n + m / nn mn.
Note that while the original bound is not symmetric in n and m, the optimal bound is.
Returning to the original notation C(r, k), let’s see how tight the optimal bound is by plotting, as a function of r, the maximum relative error as a k varies.
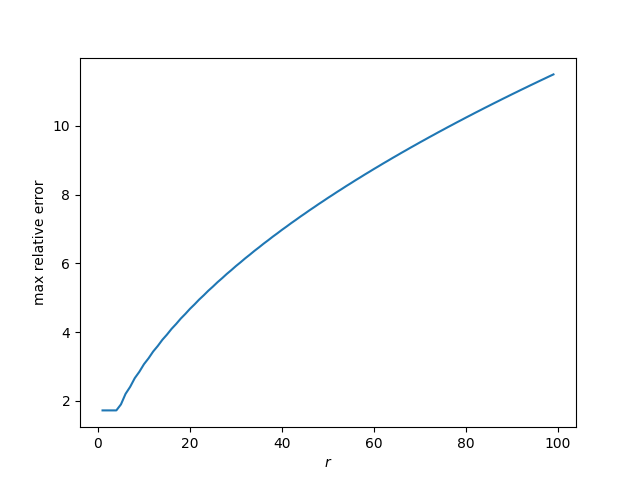
The maximum relative error, over the range plotted, is very roughly r/10.
Related posts
- Bounds on the central binomial coefficient
- Non-integer binomial coefficients
- q-binomial coefficients
[1] Grzegorz Łysik. The ε-binomial inequality. The Mathematical Gazette. Vol. 92, No. 523 (March 2008), pp. 97–99
The post Binomial bound first appeared on John D. Cook.