There is a simple rule of thumb for converting between (circular) trig identities and hyperbolic trig identities known as Osborn’s rule: stick an h on the end of trig functions and flip signs wherever two sinh functions are multiplied together.
Examples
For example, the circular identity
sin(θ + φ) = sin(θ) cos(φ) + cos(θ) sin(φ)
becomes the hyperbolic identity
sinh(θ + φ) = sinh(θ) cosh(φ) + cosh(θ) sinh(φ)
but the identity
2 sin(θ) sin(φ) = cos(θ − φ) − cos(θ + φ)
becomes
2 sinh(θ) sinh(φ) = cosh(θ + φ) − cosh(θ − φ)
because there are two sinh terms.
Derivation
Osborn’s rule isn’t deep. It’s a straight-forward application of Euler’s theorem:
exp(iθ) = cos(θ) + i sin(θ).
More specifically, Osborn’s rule follows from two corollaries of Euler’s theorem:
sin(iθ) = i sinh(θ)
cos(iθ) = cosh(θ)
Why bother?
The advantage of Osborn’s rule is that it saves time, and perhaps more importantly, it reduces the likelihood of making a mistake.
You could always derive any identity you need on the spot. All trig identities—circular or hyperbolic—are direct consequences of Euler’s theorem. But it saves time to work at a higher level of abstraction. And as I’ve often said in the context of more efficient computer usage, the advantage of doing things faster is not so much the time directly saved but the decreased probability of losing your train of thought.
Caveats
Osborn’s rule included implicit expressions of sinh, such as in tanh = sinh / cosh. So, for example, the circular identity
tan(2θ) = 2 tan(θ) / (1 − tan²(θ))
becomes
tanh(2θ) = 2 tanh(θ) / (1 + tanh²(θ))
because the tanh² term implicitly contains two sinh terms.
Original note
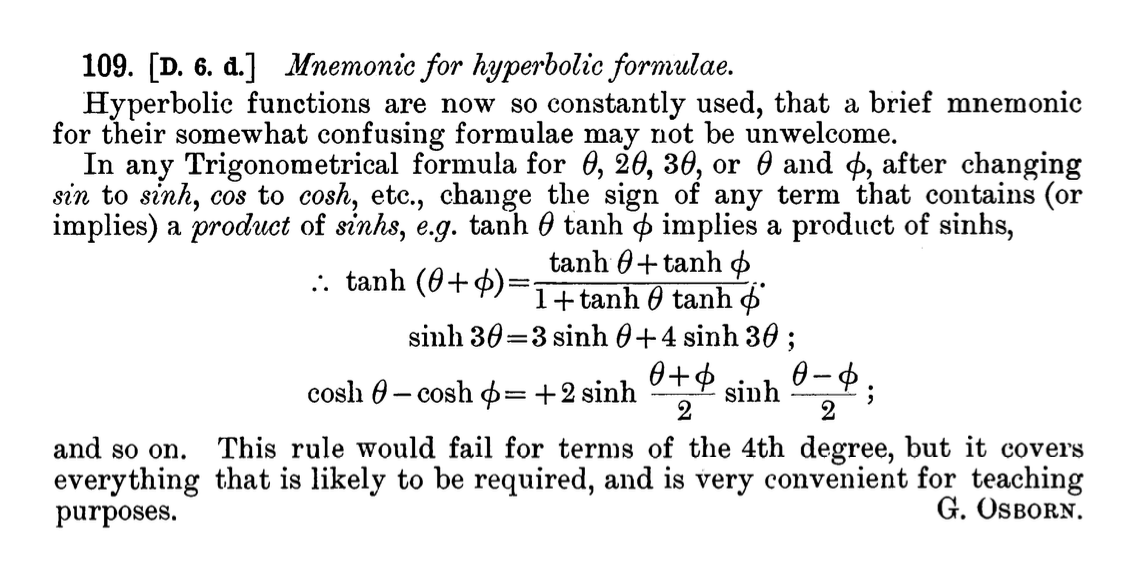
Osborn’s original note [1] from 1902 is so short that I include the entire text below:
Related posts
[1] G. Osborn. Mnemonic for Hyperbolic Formulae. The Mathematical Gazette, Vol. 2, No. 34 (Jul., 1902), p. 189
The post Rule for converting trig identities into hyperbolic identities first appeared on John D. Cook.